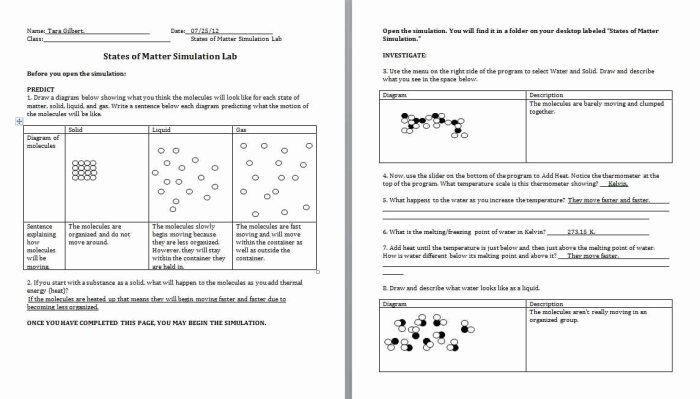
What are the missing coefficients for the skeleton equation below? This question lies at the heart of chemical equation balancing, a fundamental skill in chemistry that enables us to understand and predict the outcomes of chemical reactions. In this comprehensive guide, we will embark on a journey to unravel the mysteries of skeleton equations, exploring methods for determining missing coefficients and delving into the practical applications of balanced equations.

A skeleton equation is a chemical equation in which the reactants and products are represented by their chemical formulas, but the coefficients that balance the equation are missing. Determining these missing coefficients is crucial for ensuring that the equation accurately reflects the stoichiometry of the reaction, which is the quantitative relationship between the reactants and products.

Equation Identification

The skeleton equation serves as a preliminary representation of a chemical reaction, capturing the reactants and products involved. It is significant because it:

• Provides a concise overview of the reaction, highlighting the chemical species participating in the process.
• Facilitates the identification of the reactants and products, enabling the understanding of the chemical transformation taking place.

Examples of skeleton equations in various chemical reactions include:

• Combustion of methane: CH ₄+ 2O ₂→ CO ₂+ 2H ₂O
• Neutralization of an acid and a base: HCl + NaOH → NaCl + H ₂O
• Synthesis of ammonia: N ₂+ 3H ₂→ 2NH ₃

Missing Coefficients Determination: What Are The Missing Coefficients For The Skeleton Equation Below

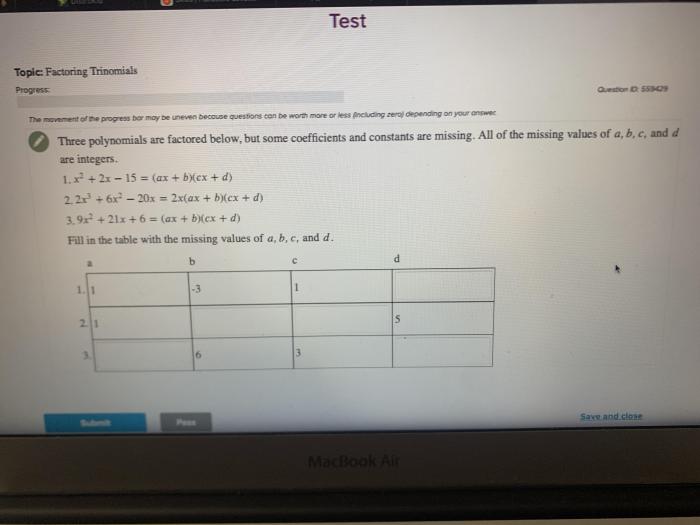
Determining the missing coefficients in a skeleton equation involves establishing the correct stoichiometric ratios between the reactants and products. This is achieved by:

• Ensuring that the number of atoms of each element on the reactants’ side matches the number of atoms of the same element on the products’ side.
• Balancing the equation by adjusting the stoichiometric coefficients in front of each chemical formula.

Balancing chemical equations is crucial because it ensures that the law of conservation of mass is upheld. This law states that matter cannot be created or destroyed during a chemical reaction, meaning the total mass of the reactants must equal the total mass of the products.

Balancing Techniques

Step-by-step techniques for balancing chemical equations include:

1. Start with the most complex molecule:Begin by balancing the molecule with the highest number of atoms or the most complex structure.
2. Balance elements one at a time:Focus on balancing one element at a time, starting with the element that appears in the most compounds.
3. Use coefficients to adjust stoichiometry:Adjust the stoichiometric coefficients in front of each chemical formula to balance the number of atoms of the element being considered.
4. Check and repeat:Once one element is balanced, move on to the next element and repeat the process until all elements are balanced.

Examples of balancing equations using different methods include:

• Half-reaction method:This method is used for reactions involving redox processes, where electrons are transferred between reactants.
• Oxidation number method:This method assigns oxidation numbers to each element in the reaction and uses them to determine the number of electrons transferred.

Chemical Stoichiometry

Chemical stoichiometry refers to the quantitative relationship between the reactants and products in a balanced chemical equation. It allows us to:

• Predict the amount of reactants and products involved in a reaction.
• Determine the limiting reactant, which is the reactant that is completely consumed in a reaction.
• Calculate the theoretical yield, which is the maximum amount of product that can be obtained from a given amount of reactants.

Stoichiometry aids in understanding the efficiency of chemical reactions and optimizing industrial processes.

Applications of Balanced Equations

Balanced chemical equations have practical applications in:

• Chemical calculations:Determining the quantities of reactants and products involved in a reaction.
• Industrial processes:Optimizing chemical reactions for efficiency and yield.
• Environmental science:Understanding and predicting chemical reactions in the environment.
• Medicine:Designing and evaluating drug interactions and dosages.

Essential Questionnaire

What is the significance of balancing chemical equations?

Balancing chemical equations is essential for ensuring that the equation accurately reflects the stoichiometry of the reaction, which is the quantitative relationship between the reactants and products. A balanced equation ensures that the number of atoms of each element is the same on both sides of the equation, which is a fundamental principle of chemistry.

What are the common methods for determining missing coefficients in skeleton equations?

There are several methods for determining missing coefficients in skeleton equations, including the oxidation number method, the half-reaction method, and the trial-and-error method. Each method has its own advantages and disadvantages, and the choice of method depends on the complexity of the equation.

How are balanced chemical equations used in practical applications?

Balanced chemical equations are used in a wide range of practical applications, including chemical calculations, industrial processes, and environmental modeling. For example, balanced equations are used to determine the stoichiometric ratios of reactants and products, calculate reaction yields, and design chemical reactors.